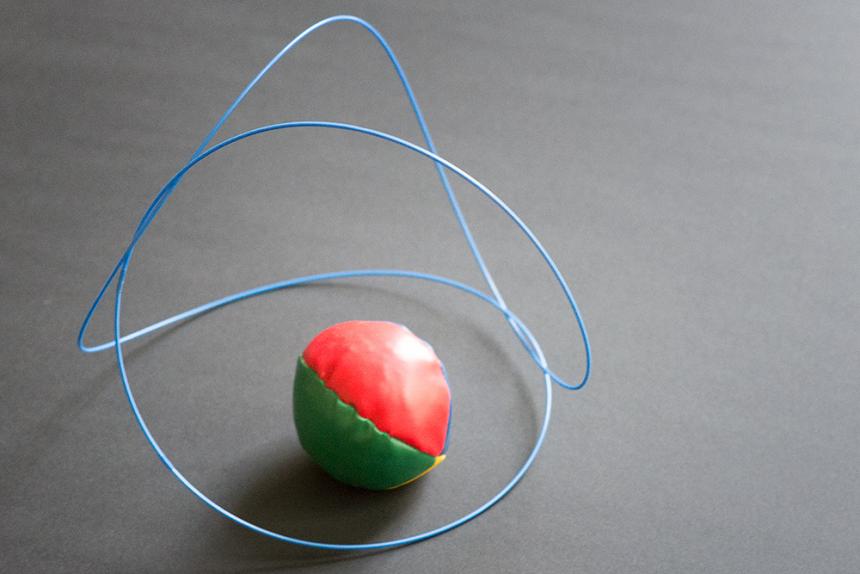
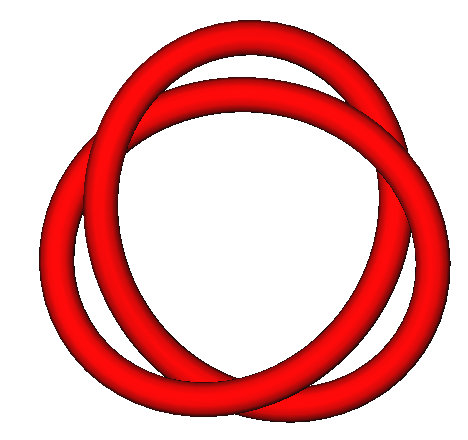
... a knotted springy wire cannot rest in stable equilibrium without points of self-contact—an experimentally observable fact. This fact leads to a rather curious “topologically constrained” variational problem; what actually happens if one forms a knot in a piece of springy wire? Experiments yield some beautiful curves with impressive symmetry ...
Langer and Singer's question [LS] leads to a free obstacle problem that involves techniques at the interface of geometric analysis, low-dimensional topology, modeling, numerical analysis, and nonlinear optimization.
Elastic energy
Neglecting all effects of twist and shear, we consider a simplistic model by assuming that the shape of the wire is only influenced by the bending energy of its centerline \(\gamma:\mathbb{R}/\mathbb{Z}\to\mathbb{R}^3\). The latter amounts to \[ E_{\mathrm{bend}}(\gamma) = \int\limits_{\mathbb{R}/\mathbb{Z}}\kappa_\gamma(s)^2\;\mathrm d s \] where \(\kappa_\gamma(s)\) denotes the scalar curvature at \(\gamma(s)\) and \(s\) is an arc-length parameter.
The model
In order to model the impermeability of the curve, we regularize the bending energy by a functional \(\mathcal{R}\) that models self-avoidance. This means that its values blow up on sequences of embedded curves converging to a curve with a self-intersection. There are several candidates for this purpose.
As proposed in [vdM], we study the problem of minimizing the total energy \[ E_\vartheta = E_{\mathrm{bend}} + \vartheta \mathcal{R} \] within a given knot class. We expect that the limit of \(E_\vartheta\) minimizers as \(\vartheta\searrow0\) does not depend on the choice of \(\mathcal{R}\).
Employing the ropelength functional, i.e., the quotient of length over thickness, reflects the idea of a tube with a uniform radius. Thickness of a curve can be defined as the infimum over the radii of all circles passing through three distinct points of the curve [GM].
Two-bridge torus knots
In case of the trefoil knot class, minimizers of \(E_\vartheta\) tend to the doubly covered circle as \(\vartheta\searrow0\) [GRvdM]. This statement also applies to any \((2,b)\) torus knot class where \(b\ge3\) is an odd number. This proves a conjecture of Gallotti and Pierre-Louis [GPL] for two-bridge knots.The proof relies on a generalization of the Fáry–Milnor theorem [M] to the \(C^1\) closure of a knot class which in turn bases on the existence of alternating quadrisecants for knotted curves due to Denne [D].
References
| [D] | Elizabeth Denne. Alternating quadrisecants of knots. Doctoral dissertation, University of Illinois at Urbana-Champaign, 2004. [ arXiv ]. |
| [GPL] | Riccardo Gallotti, Olivier Pierre-Louis. Stiff knots. Phys. Rev. E (3), 75(3):031801, 2007. [ arXiv | doi ] |
| [GRvdM] | Henryk Gerlach, Philipp Reiter, and Heiko von der Mosel. The elastic trefoil is the doubly covered circle. Arch. Rat. Mech. Anal., 225(1):89–139, 2017. [ arXiv | doi ] |
| [LS] | Joel Langer, David A. Singer. Curve straightening and a minimax argument for closed elastic curves. Topology, 24(1):75–88, 1985. [ open archive ] |
| [M] | John W. Milnor. On the total curvature of knots. Ann. of Math. (2), 52:248–257, 1950. [ doi ] |
| [vdM] | Heiko von der Mosel. Minimizing the elastic energy of knots. Asymptot. Anal., 18(1-2):49–65, 1998. [ preprint | journal ] |